To download the below  and all other Useful Books and calculations Excel sheets please click here 

To download the below  and all other Useful Books and calculations Excel sheets please click here 

Calculation of raw mix composition

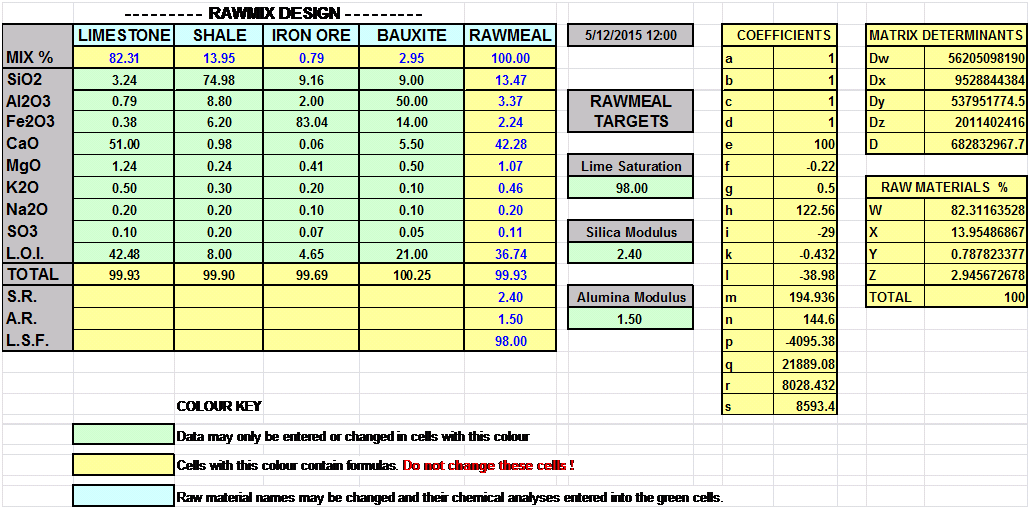
raw mix design calculation pdf
raw mix design excel sheet
cement raw mix calculation
cement raw mix chemical composition
cement raw mix design software
raw mix design cement manufacturing
raw mix to clinker ratio
clinker factor calculation

The purpose of calculating the composition of the raw mix is to determine the quantitative proportions of the raw components, in order to give the clinker the desired chemical and mineralogical composition. For this, there are many methods of calculation; from the most simple to the more complicated ones. The basis for calculation is the chemical composition of the raw materials. Generally, data of chemical  analyses should be accurate to two decimals. Results of analy­ses in excess of 100 %, should be arithmetically reduced to 100 %, each constituent being proportion­ ally reduced. If on the other hand the total of the con­stituents is less than 100, the constituents are not pro­ portionally increased to 100; in this case the differ­ence from 100 is denoted as “rest”, so that the total of all constituents is then 100.

Alligation alternate method

The simplest course of calculation for solving blend­ing problems is known as alligation alternate; this method allows the determination of the proportion of two raw material components. In this case, only the required lime content is fixed as a setpoint, so that the proportion of both components can be deter­mined.

Example 2.1.

What mixing proportion is required for limestone with 91 % CaC03, and clay with 31 % CaC03, to get a raw mix with a CaC03 content of 76 %? According to alligation alternate, the procedure is as follows:

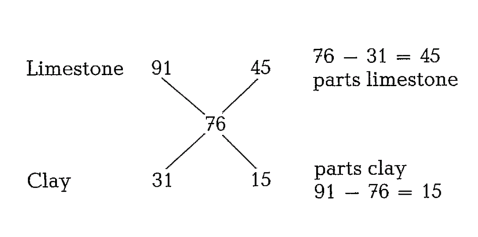
To get a raw mix with a CaC03 content of 76 %, 45 parts of limestone should be mixed with 15 parts of clay. Thus the proportion of the components in the raw mix, i. e. limestone : clay = 45 : 15, or 3 : 1.

Calculation based on the hydraulic module

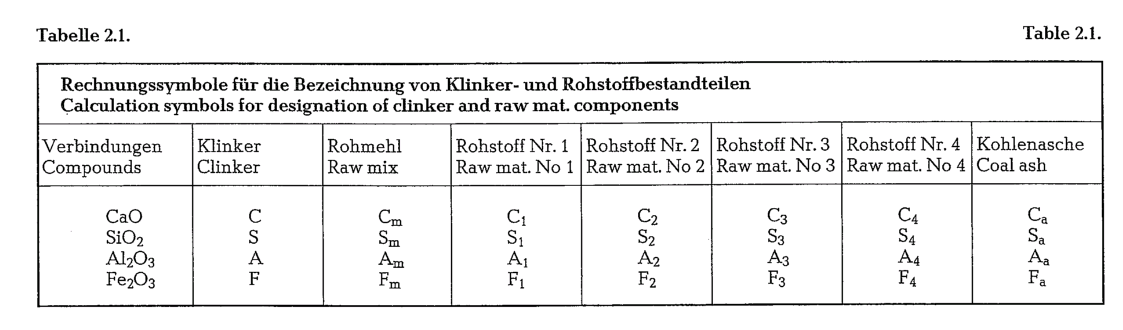
This method is applicable to two raw material compo­nents, with the hydraulic module selected for the clinker [15, 16]. To simplify the following calculations, symbols are used for the designation of the clinker components, the raw materials, and the coal ash; these symbols are placed in table 2.1.

Applying  these  symbols,  the  hydraulic  moduli  for clinker and raw mix will be:

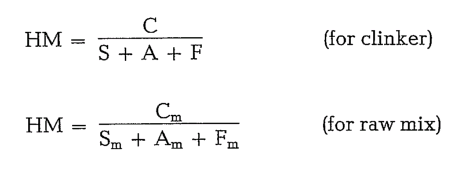
Since both moduli  are of  the same value, one can equate:

According to this method of calculation it is assumed that x parts of the first raw material are apportioned to one part of the second raw material. Under this assumption, the quantities of  the particular raw material components can be calculated by using the following formulas:

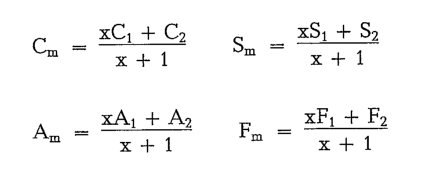
Inserting the values Cm, Sm. Am and Fm into the for­mula for the hydraulic module, we get

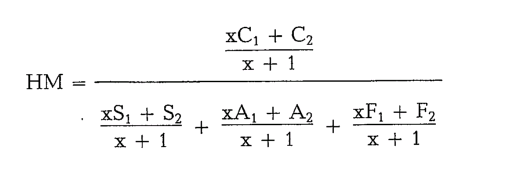
Since the oxide ·components are known from the chemical analysis of  the raw materials, and the hydraulic module is selected according to the quality requirements, the only remaining unknown is x. After transformation of the above formula, to calcu­ late the value for x, the following formula appears:

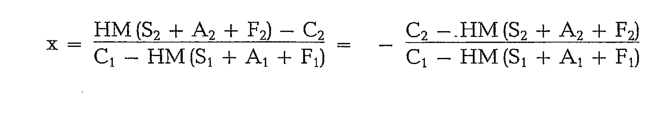
Example 2.2.

Two raw materials are given with the following com­ position (see column 1 and 2 of table 2.2.)

Calculate the composition of the raw mix, assuming a hydraulic module of HM = 2.2. Solution:

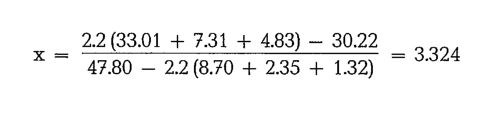
i.e. to get a clinker with a HM = 2.2, we have to mix 3.324 parts limestone with one part of marl.

Thus the raw mix consists of:

76.87 % limestone, and

23.13% marl.

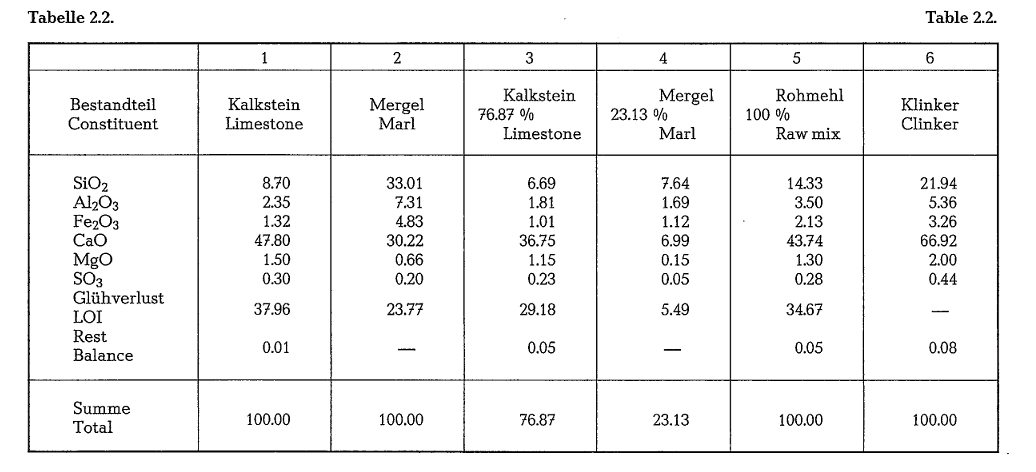
In  table  2.2.  the  calculated  raw  mix  components appear in column 3 and 4, and the composition of the

raw  mix  is  given  in  column  5 (columns  3  + 4  =column 5). Column 6  contains  the  calculated  clinker

composition as raw mix of column 5, free of loss on ignition. From column 6 we obtain the value for the

hydraulic module HM = 2.2.

Calculation based on lime saturation factor

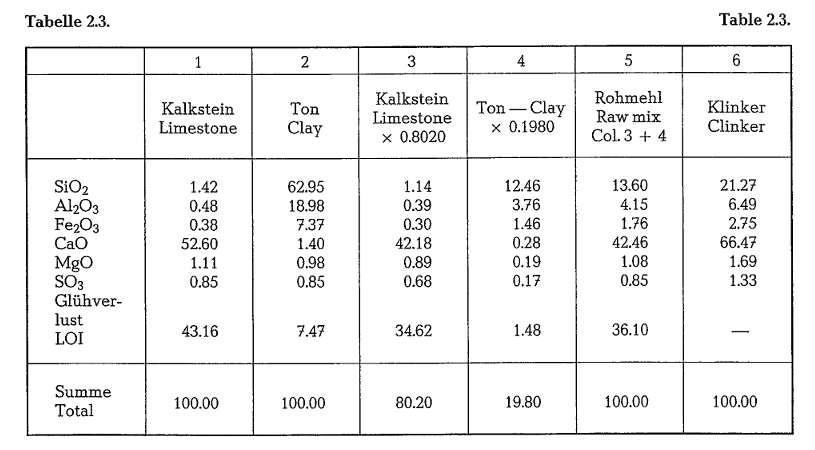
Example 2.3.

Given are 2 raw materials (see table 2.3., column 1 and 2); Kind’s lime saturation module is:

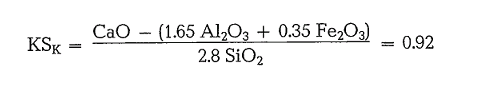
Inserting into Kind’s formula the calculation symbols used previously, we get:

and solving for x:

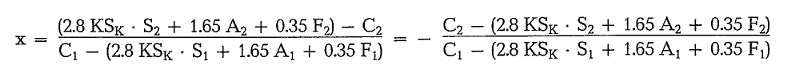
With this formula we calculate how many parts of limestone in the raw mix are apportioned to one part of clay. Accordingly we get:

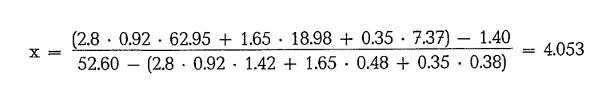
Thus  4.053  parts limestone  are  apportioned  to  one part of clay, and the raw mix consists of

80.20 % limestone, and 19.80 % clay.

Columns 3, 4, 5, and 6, of table 2.3. contain the calcu­ lated parts of components, as well as the raw mix and clinker composition. The resulting  lime  saturation factor is:

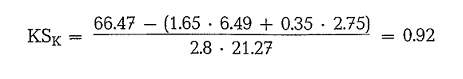
This proves the accuracy of the calculation.

Calculation with lime saturation factor and silica ratio

Example 2.4.

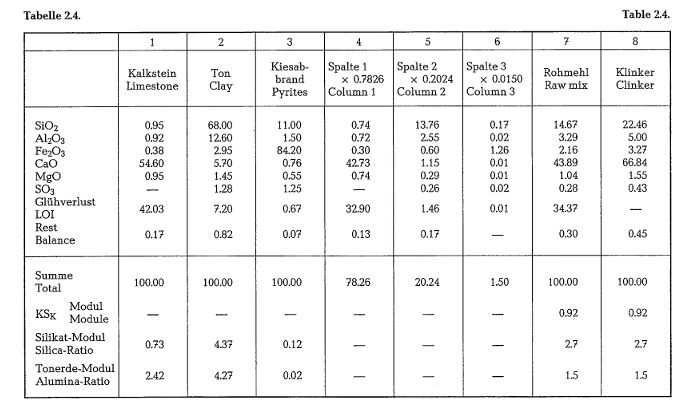
Calculation of a raw mix by. the aid of three raw materials; the  required  Kind’s  lime  saturation  factor is 0.92, and the required silica ratio is 2.70. The analy­ ses of raw materials are shown in column 1, 2 and 3, of table 2.4.

Solution:

The formula for Kind’s lime saturation factor was already outlined in example 2.3. Applying the sym­ bols used previously, the formula for the silica ratio reads:

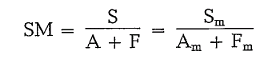
The calculation is performed under  the  assumption that x parts by weight of  limestone  (component 1), and y parts by weight of clay (component 2), are apportioned to one part by weight of pyrites cinders (component 3). Then the following formulas apply to the oxide components of the raw mix [19]:

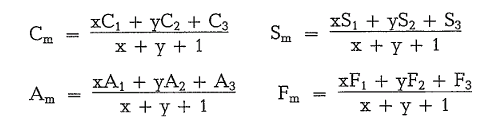
Inserting the above expressions into the formula for the lime saturation factor, and into the formula for the silica ratio, we get two equations with two unk­nowns x and y; and after transformation we get:

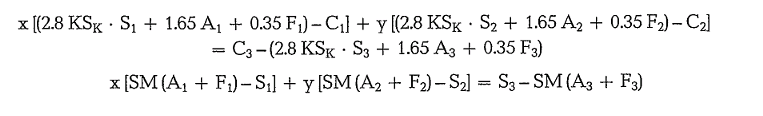
For a better review, the following additional abbrevia­tions or symbols are applied:

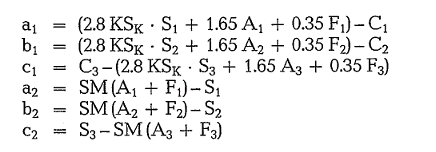
When   employing   these   symbols,   the   equations quoted above will take the following form:

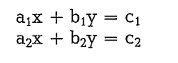
After solution of these equations, we get the values for x andy

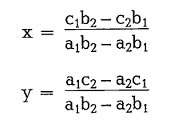
To calculate the alumina ratio instead of the  silica ratio, i. e. to calculate the  lime  saturation  factor  and the alumina ratio, a2, b2, and c2, will take the following values (a1, b1, and c1, remain unchanged):

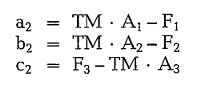
To calculate the parts of limestone x, and of clay y, which are apportioned to one part of pyrites cinders, the above developed formulas for x and y should be used.

First the values for a1, b1, c1, as well as for a2, bz, and c2, must be calculated.

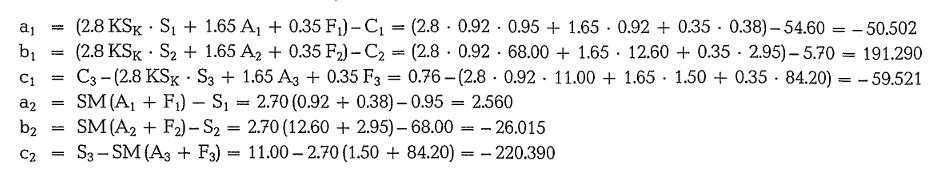
Now, the resulting values are inserted into the formu­ las for x and y:

The result is that 53.03 parts of limestone and 13.69 parts of clay are apportioned to 1 part of pyrites cinders, or that the raw mix will consist in percent of:

The calculated figures of columns 4, 5, 6, 7, and 8, of the table 2.4., prove the correctness of the method of calculation.

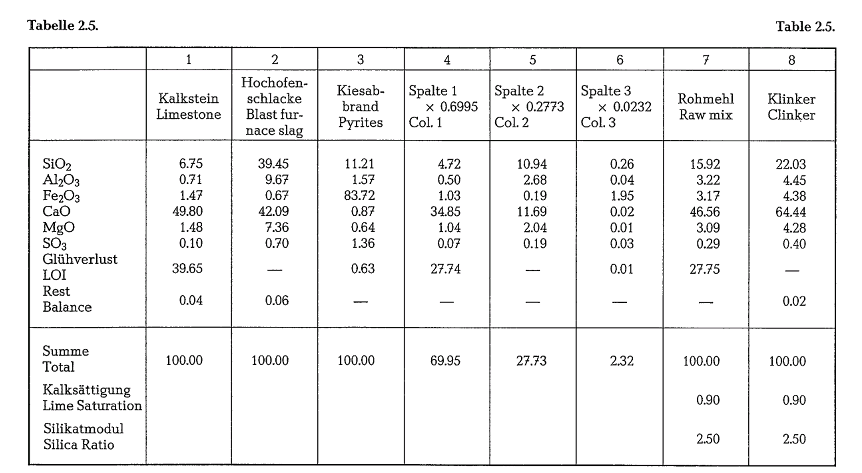
Example 2.5.

Calculation of a raw mix consisting of three compo­ nents: limestone, blast furnace slag, and pyrites cin­ ders. The required lime saturation factor is 0.90 and the silica ratio 2.5. The analyses of the raw materials are shown in columns 1, 2, and 3 of table 2.5.; columns 4,  5,  6,  7,  and  8,  of  this  table  contain  the  calculated results.

The course of  calculation is the same as for example 2.4.

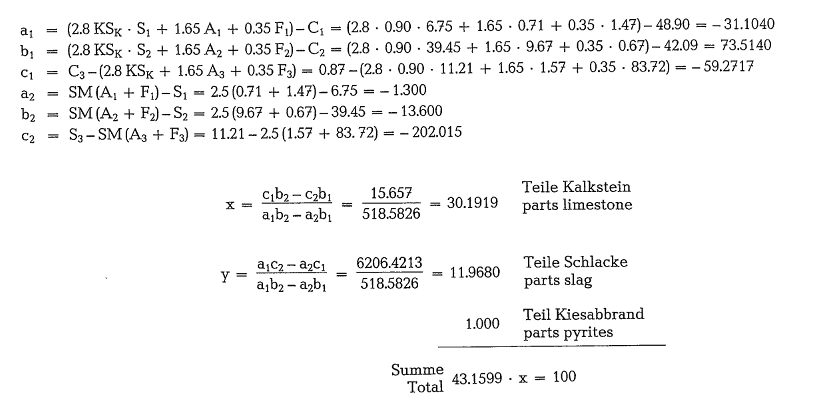
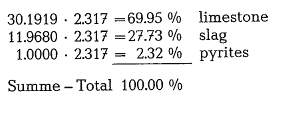
The figures in columns 4, 5, 6, 7, and 8, of table 2.5., prove the correctness of the calculation.

Calculation of the quantity of coal ash absorbed by the clinker

There is no absorption of fuel ash by the clinker, when using natural gas or fuel oil for clinker burning. On the contrary, when using coal, the matter of coal ash absorption by the clinker should be taken into account.

In modern long rotary kilns as well as in preheater kilns, i. e. in all kilns where dust losses are almost eli­ minated, the ash absorption by the clinker is total. Short rotary kilns with high dust losses, show a low degree of ash absorption. Generally, the ash absorp­ tion rate by the clinker in rotary kilns is in the range from 30 to 100 %, depending upon the kiln type. Shaft kilns for clinker burning always show an ash absorp­ tion rate of 100 %. For a certain kiln type, the ash absorption rate is constant.

Example 2.6.

The ash absorption rate can be calculated by using the analyses of the raw mix, the clinker, and the coal ash. The symbols for the particular components and oxides are the same as specified above. The rate of ash – absorption in per cent of the clinker weight is denoted by q; then we get the following equations [24]:

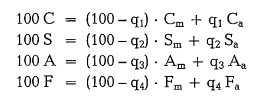
Solving these equations for q, we get the following expressions:

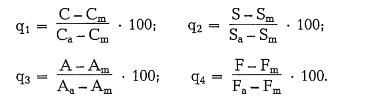
The arithmetical mean of q1 to q4 produces the desired value for q. The raw mix being specified as free of loss on ignition, the chemical analyses of the raw mix, the clinker and the coal ash are shown in table 2.6.

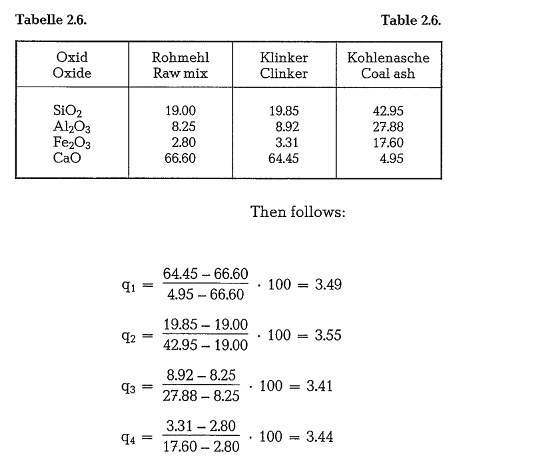
and the arithmetic mean

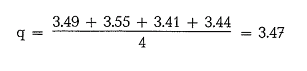
That is to say that the quantity of coal ash absorbed by the clinker during the burning process is 3.47% of the clinker weight.

Example 2.7.

Here, another method is presented to  calculate the influence of the coal ash on the chemical composition of clinker.

The chemical composition of the coal ash is:

The composition of the clinker is:

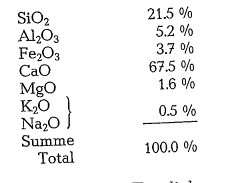
The clinker composition without coal ash is:

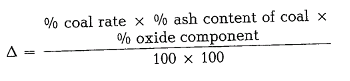
It is assumed that the coal rate is 19 % of the clinker weight; the ash content of the coal is 12 %, and the ash absorption rate is 100 %. Then the ash compo­nents in the clinker are:

The corrected  clinker composition is shown in table 2.7.

The  modules  of  the  ignited  raw  mix  (column  5,  of table 2.7.), are:

These moduli allow calculation of proportioning of a raw mix which consists of two or more components, using  the proper  calculation  method  applicable  for multicomponent raw mixes. Clinker burned from raw mix which was calculated as shown, will then have the composition as specified in column 2 of table 2.7.

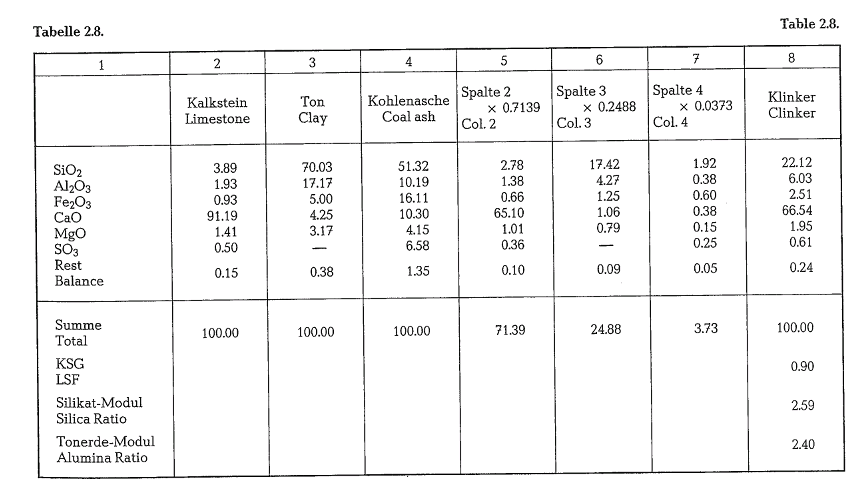
Example 2.8.

Calculate a raw mix consisting of two components, limestone and clay, considering the influence of the coal ash on the composition of the clinker; the lime saturation factor KSK (according to Kind), is assumed to be 0.90.

The chemical composition of these three raw material components is as shown in columns 2,  3,  and  4,  of table 2.8. [19].

The coal consumption is 35 % referred to the clinker weight; the ash content of the coal is 16.4 %; and the ash absorbed by the clinker is 65 %. Then the amount of ash embodied in the clinker comes to

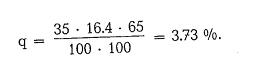
Now, the values for at, bt, Ct, and for a2, b2, c2, are cal­ culated.

For this calculation it is assumed that x parts of lime­ stone, plus y parts of clay (ignition free) plus q parts of ash amount to 100 parts of clinker. Thus:

X+ y + q = 100

Applying the symbols shown in table 2.1., the oxides in the clinker can be expressed by the following equations:

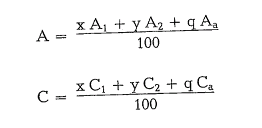
If the above values are inserted into the lime satura­ tion factor for the clinker, then we get the following equation with 2 unknowns:

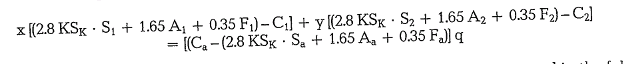
The equation can be expressed in the following form

When using the following abbreviations, we get:

To  calculate  x  and y,  the  following  two  equations should be solved:

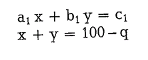
To get formulas similar to that wich were used in the previous calculations, the following abbreviations should be applied for the second equation:

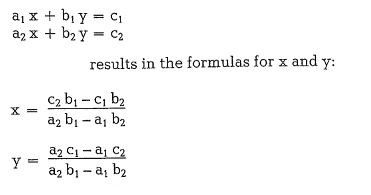
The solution of the following two equations

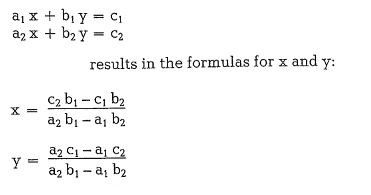
Now, the example is calculated as follows:

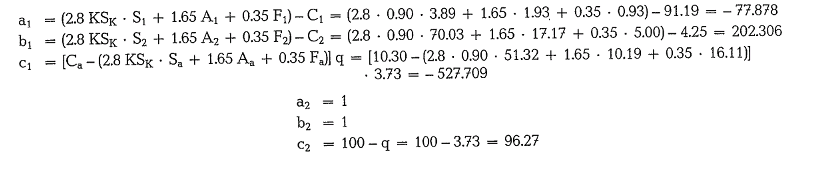
Inserting the above values into the formulas for x and y, we get after conversion:

From the calculation results that 71.39 % limestone (ignited), 24.88 0/o clay (ignited), and 3.73 0/o coal ash participated  in the clinker formation.

The computed chemical composition of the clinker is shown in columns 5, 6, 7, and 8, of table 2.8. As is shown by the calculation, the lime saturation factor is 0.90, i. e. as much as assumed for the calculation. This demonstrates the correctness of the calculation. For the practical completion of the proportioning of the raw materials, it is required to convert them to the unignited basis. This is performed as follows:

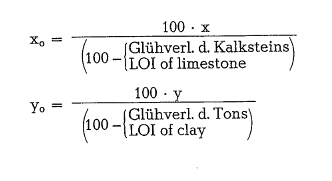
Calculation with four raw material components

The following example contains formulas to calculate a raw mix consisting of four raw material compo­nents, with selected values for the lime saturation factor, the silica ratio and the alumina ratio.

Example 2.9.

It is assumed that the ratio of the raw material com­ ponents is:1.component : 2. component : 3. component : 4. com­ ponent = x : y : z : 1; then the oxide formulas are inserted into the formulas for the lime saturation fac­ tor, the silica ratio, and the alumina ratio. The result­ ing three equations are then converted into the sim­ plified form of three linear equations with three un­ knowns; consequently we get:

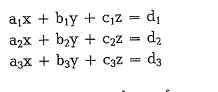
where  for  reasons  of   simplification  the  following notation is used

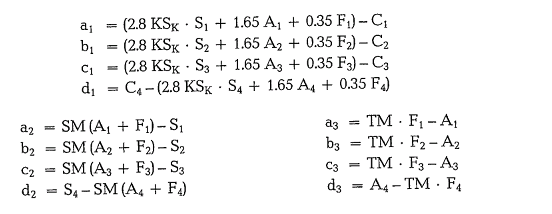
After solving the above equations for x, y, and z, we get the following formulas for the computation of a raw mix composed of  four raw material components [25]:

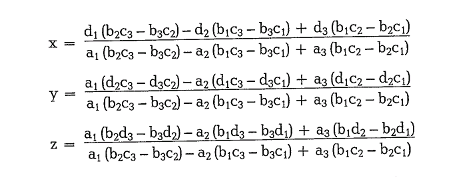
Raw mix calculation to find the required amount of potential clinker compounds

The following demonstrates a calculation for the determination of the proportions of the raw material components to be used for producing a clinker of a required potential composition of minerals.

Example 2.10.

Given are two kinds of raw materials, limestone and clay, the chemical composition of which is shown in table 2.9., column 1, and 2. Columns 3, 4, 5, and 6, con­ tain the calculated composition of the clinker and the potential phase composition. Using the formulas in section 1.6. the four main minerals of the  two raw materials,  i.e. limestone  and clay (ignition free), can be calculated. Table 2.10. contains the computed com­ position of the clinker minerals. The next step is to mix the limestone and clay in such a ratio that the blend of both components will result in the required content of tricalcium silicate, C3S, of 60% [12].

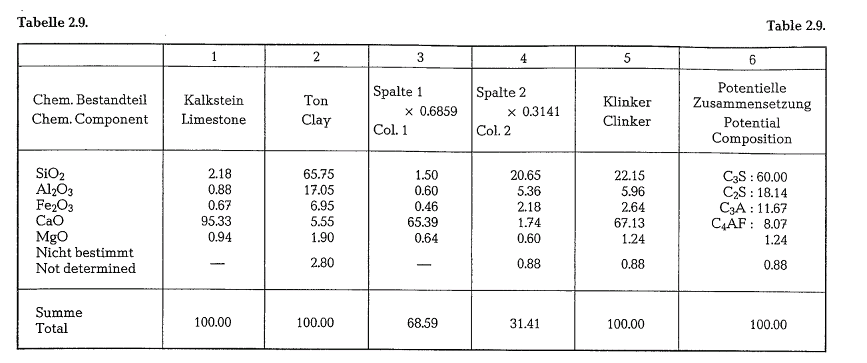
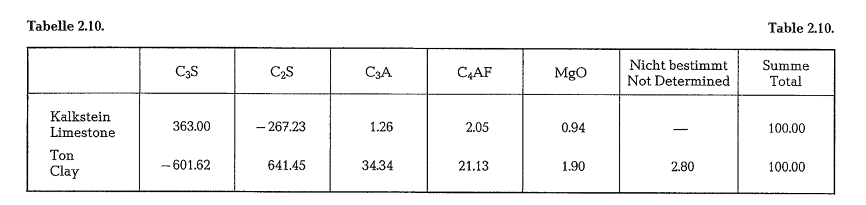
be calculated. Table 2.10. contains the computed com­position of the clinker minerals. The next step is to mix the limestone and clay in such a ratio that the blend of both components will result in the required content of tricalcium silicate, C3S, of 60% [12].

This ratio is found with the help of the following for­mula:

In the formula x is the part of the second component (clay); A is the percentage of the desired mineral in component No.1 (limestone), B is the percentage of this mineral in component No.2 (clay), and R is the percentage of this mineral in the blend of these two components. Table 2.11. contains the calculated parts by weight of limestone and clay, which when blended, produce a raw mix or clinker with the required content of 60% C3S. For this mixture, it may be seen that 68.59 % limestone, and 31.41 % clay are necessary.

Example 2.11.

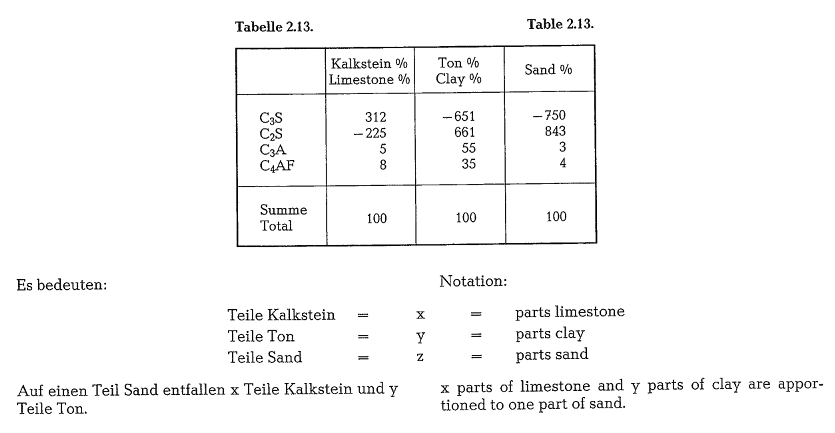
Calculation of a raw mix consisting of three compo­nents (limestone, clay, and sand), with specified con­ tents of tricalcium silicate, and dicalcium silicate.

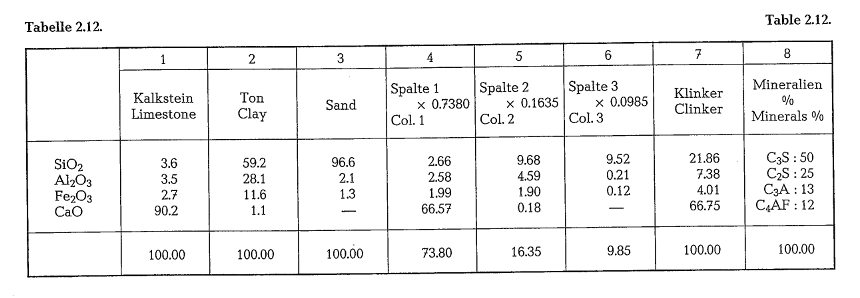
For this, the calculation with determinants may be applied.

Columns 1, 2, and 3, of table 2.12., show the composi­ tion of  the  raw  materials.  The  required  raw  mix should contain 50 0/o C3S, and 25 0/o C2S.

The calculated potential mineral composition of the three components is shown in table 2.13.

To solve this problem, we first assemble three linear equations as in example 2.9.:

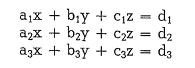
and  solve for x, y, and  z, using  third-order  determi­nants [26, 5]:

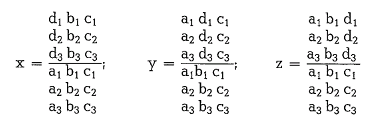
As is well known, the solution involves multiplication of three elements located on a diagonal, as shown here schematically. For clarity, the first two columns are extended by repeating them on the right side.

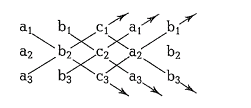
As is known, if we multiply downwards, the products are added; and if we multiply upwards, the products are subtracted, as follows:

Now,  we  insert  into  the  equations  the  required amount of minerals of the components

The value for z is being found by difference:

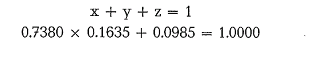
The percentages of the three components are (igni­tion free).

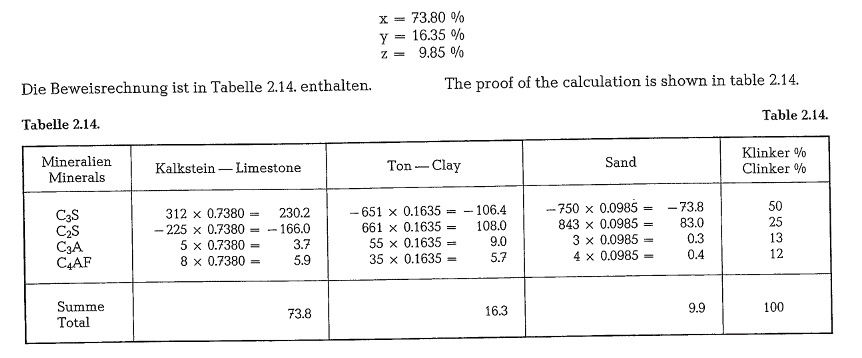
The calculated composition of  the  clinker  as well  as the conventionally calculated  content  of  clinker minerals in round figures, are shown in columns  4, 5, 6, 7, and 8, of table 2.12.

Suppose that instead of 50 0/o C3S we insert into the above calculation, values of 25 % C3S, and 50 0/o C2S; we then get the  following  percentages  of  raw  mate­rial  components  (ignition  free):

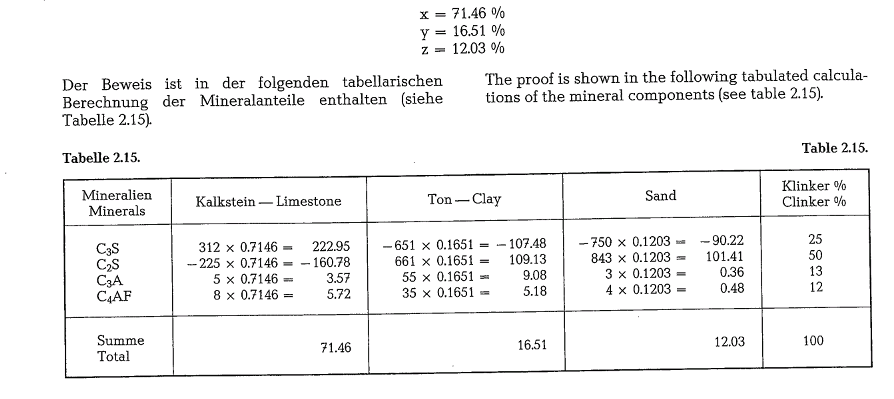
Oxide contents and potential composition

The above calculation shows that small quantity fluc­tuations in the proportioning of the raw components, with their resultant small differences in the oxide contents, cause large fluctuations in the potential phase composition of the clinker and consequently,as in this case, the reduction of C3S-content by 50%, and an increase in C2S-content of 100 %. This is also shown in the following tabulated compilation of three different clinkers with small differences in the oxide contents, and the resulting large fluctuations in the calculated phase composition (see table 2.16.) [27].

Table 2.16. contains chemical compositions of three different clinkers. The oxide contents do not vary widely; but the calculated potential compositions show considerable differences. Therefore, when cal­ culating the composition of the raw mix, it should be taken into consideration that a variation of 1 % in the CaO-content causes a variation in the tricalcium sili­ cate content by about 10-14% and, vice versa, a fluctuation in the C3S-content by 1 %, causes a cor­ responding increase or decrease in the CaO-content by 1/14 = 0.07 %, or a change in the CaCOTcontent of the raw mix by 0.07 x 1.78 = 0.12 %. If the CaO­ content is kept constant and the values for the other oxides change, there is a  corresponding change in contents of the resulting clinker minerals.

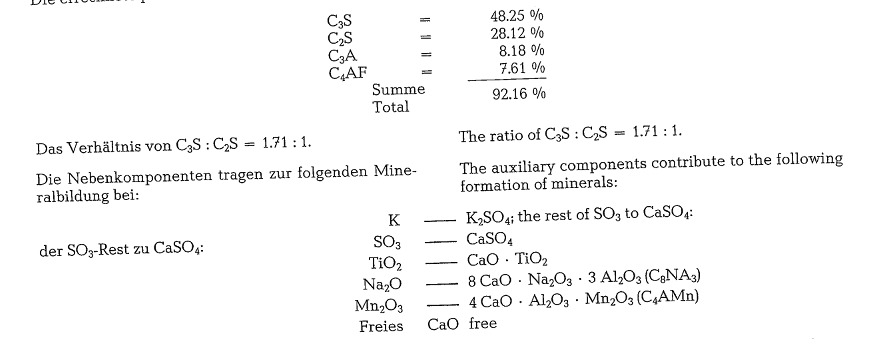
Also one and the same clinker can show different cal­ culated potential compound compositions, when  on the one hand, one calculates with only the four main oxides, and on the other hand when including also the auxiliary components which participate in the formation of minerals. For a numerical presentation of this fact, the following clinker analysis may be used for calculation [28].

The calculated potential composition is:

Considering the mineral formation  as quoted above, the potential clinker composition is:

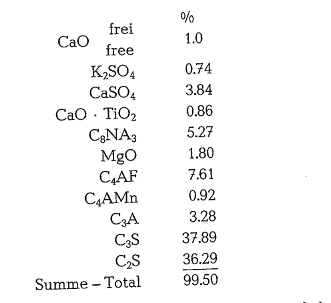
A comparison of the two results shows wide differ­ ences in the potential composition of the same clinker. Also the ratio  c3s : c2s has changed and is roughly 1 : 1, according to the second method of cal­ culation. Differences in potential composition are the result of differences in the mineral formations used for the calculation.

Download Link