click here to Download the Most Important 13 Books in Cement Industry 

click here to Download the Most Important 13 Books in Cement Industry 

EVERYTHING YOU NEED TO KNOW ABOUT OPERATION AND CONTROL OF CLINKER COOLER

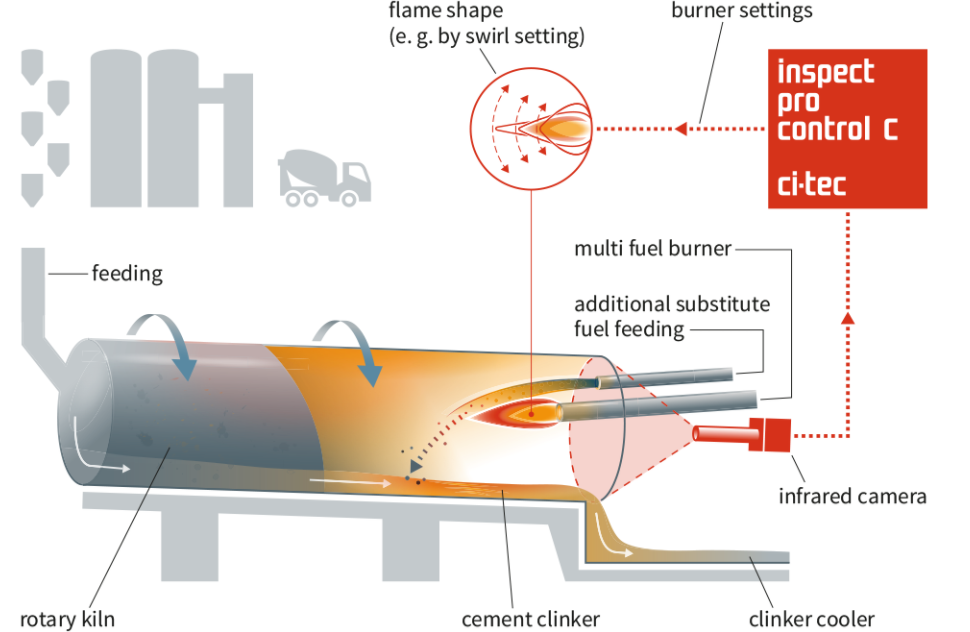
It is extremely important for the operator to master cooler controls as well as other control functions of the rotary kiln  system,  because  the cooler itself performs an integral part of the clinker-burning process. Cooler conditions influence the  burning process in the  kiln and conse­quently, the quality of the clinker. There are many instances where cooler operating problems can lead a perfectly stable kiln into a severely upset condition with possible damage to the equipment.

With the introduction of large-sized rotary kilns having production ca­pacities in excess of 1500 tons per day, traveling grate cooler.; have become a problem in some plants. Unexpectedly, widespread complaints began as cooler components burned up and entire coolers had to be repaired at alarming rates. These problems were not only experienced in North America but were also encountered throughout the world wherever large rotary kilns had been placed into service. Regrettably, a final solution to the problem has not yet been found, and in some cement plants  frequent cooler failures due to overheating still persist. On the other hand, major modifications and improvements in the design of cooler equipment in the past few years have lessened the problems to a large extent and indications are that coolers can now be made 19 operate in a satisfactory manner. Also, not all cooler failures are caused by design of the cooler itself, for inexperience and laxness on the part of operators has contributed  to the problem. The detailed description of cooler control functions in  this chapter can give the operator the information he needs to help lessen the frequency of cooler failures.

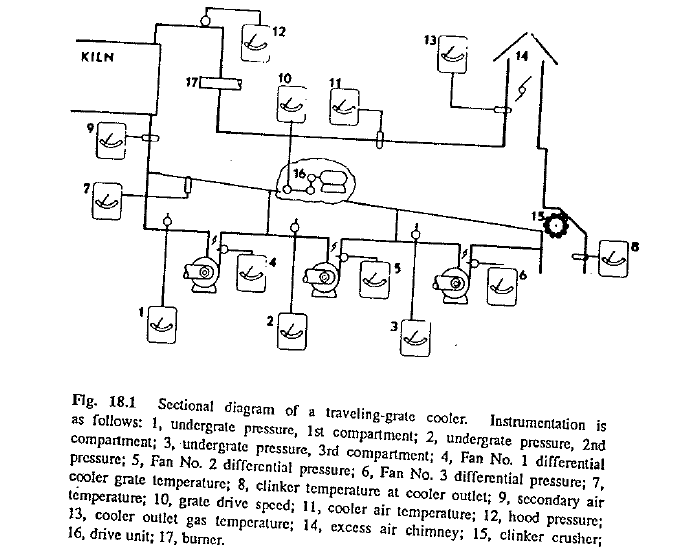
Fig. 18.1 shows a schematic layout of cooler controls to familiarize the leader with the various terms used. It does not represent any particular nake of cooler, but shows the components and  principles  involved  in cooler construction and operation.

CRITICAL VARIABLES IN COOLER CONTROL

The kiln operator must operate the cooler in such  a manner as to meet e following objectives as closely as possible:

a)Clinker temperature  at  the  discharge of  the  cooler should be as low as possible because high temperatures endanger the transport equipment and waste valuable heat.

b)Secondary air temperature should be as stable and high as possible because this  is  a prerequisite  for overall kiln  operating  stability and good fuel efficiency.

c)Cooler exit-air  temperature  should  be  as  low  as  possible  and volume  as small as possible to assure a minimal  amount of heat wasted to the atmosphere.

d)Hood pressure should always be slightly negative.

e)Depth of the clinker bed in the cooler should be such that a free passage of air through the bed can take place.

f)Cooler control settings should be such that bed grates, cooler drive unit. clinker crusher, and cooler walls cannot become overheated.

The kiln operator has basically two control variables for accomplishing the above-mentioned objectives: the speed of the bed grates which alters the clinker residence time and the clinker bed depth in the cooler, or the air distribution in the cooler can be changed.

In case of an emergency, such as badly overheated cooler conditions, the kiln operator has two more possibilities to bring the cooler under control: the amount of clinker falling into the cooler can be decreased by slowing tl1e kiln speed, or the temperature of the clinker falling into the cooler can be lowered by adjusting the flame geometry to shift the burning zone further back in tile kiln.

Numerous indicators and recorders on the plant control panel provide the means by which the operator maintains surveillance  over operation of the cooler and enables the detection of irregularities in operation. These in­ struments should  be  observed  and checked  on  a  regular  basis.  Those  that are most commonly used are undergrate air pressure, secondary air temper­ ature, grate speed amperage drawn by grate drive motor, and the clinker­ discharge temperature  at  ti1e  cooler   outlet.  In  addition  to  these  five essential instruments, there are other recorders that assist the operator in obtaining an overall indication of cooler performance. These additional instruments record t e grate temperature, cooler exit·air temperature or circulating air temperature, flow rate of air forced into the cooler, and quench-air temperature measured at a point midway of  the  cooler  length above the clinker bed. Other  frequently  used  instruments  are  a  nuclear gauge for measuring clinker bed depth, and a television camera and monitor showing the cooler interior.

The  discussion  that  follows  focuses  on  traveling  grate  coolers  since these are the most commonly used and the most difficult to control. This type of a clinker cooler is shown in Fig. 18.1.

UNDER GRATE PRESSURE  AND AIR-FLOW  RATE CONTROL

These two controls, undergrate pressure and air flow, are probably the most significant parts of cooler control because they constitute the key to successful achievement of the objective of cooler control. A thorough knowledge and understanding of these controls is essential to the operator to enable him to maintain operating stability of the kiln and prevent the cooler components from overheating.

Before the procedures in undergrate pressure control are explained, a very important point has to be stressed: The described procedures are only vaild when the cooler contains clinker that has been properly burned. These procedures should not be followed when unburned, dusty clinker or raw feed has entered the cooler.

The cooler system shown in Fig. 18.1 has three undergrate compart­ments, each compartment receiving cooling  air  from  an  individual  fan. The cooler bed-grate drive unit is in the center portion of the cooler. For control purposes, various instruments record the  undergrate  pressure  in each compartment, air-flow rates delivered by each fan, and the speed of the bed grates. Under normal operating conditons (stable operation), there is an undergrate pressure for each compartment that ensures proper cooling of the clinker. By holding this undergrate pressure constant, the operator will theoretically hold the cooler control fairly constant and in balance.

Undergrate pressure is governed mainly by the following factors:

1. Depth of the clinker bed over the grates
2. Average particle size of the clinker in the cooler
3. Temperature of the clinker in the cooler, and
4. Amount of air introduced into the cooler.

Thick beds have higher resistance and thus require more force from the fan to push tl1e air through than a thin bed. In other words, assuming that the other factors listed above remain constant, a thicker clinker bed results  in higher undergrate pressure.

Depth of the clinker bed can be controlled by speed of the cooler grates. When a thinner bed is required, the grate speed is increased. A deeper bed can be obtained by slowing the grate speed. Because of the relationship between undergrate pressure and bed depth, it is possible to maintain a con­stant undergrate pressure by regulating the grate speed. Some cooler installations have automatic controls that work on this principle; a set­ point is selected on the controller for a desired undergrate pressure and the grate speed is automatically adjusted whenever the pressure deviates from the setpoint.

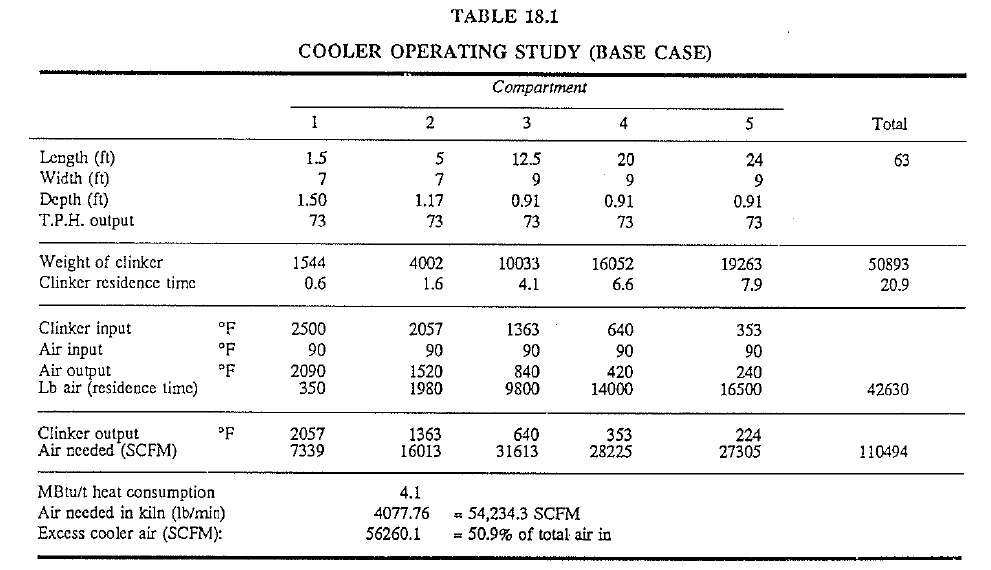
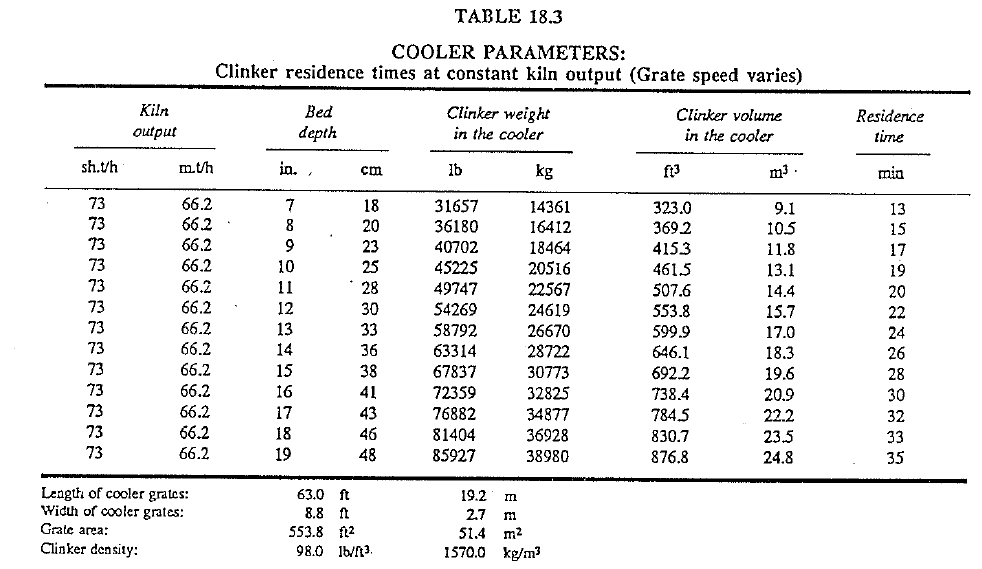
This  approach   in  cooler  control  is  usually   satisfactory  when  the  kiln operates in a stable fashion.  However, during upset kiln conditions, or when the-clinker characteristics change, this approach leaves much to be desired. During such times, an operator can often experience difficulties in maintaining  proper  cooling  of  the clinker.   For example, consider  the base operating  condition  shown  in  Table  18.1 with  a  second  compartment  bed depth of  14 in. (36 em)  at an  hourly  clinker  throughput rate of 73 tons.  In this  base case,   the clinker  residence  time in the cooler is 20.9 min  and the clinker-discharge  temperature  224  F.   Now  assume   that the kiln  suddenly starts to discharge clinker  at a rate of  89 tons/h  and that the grate speed is on automatic control, i.e., starts to increase the speed to maintain the same clinker-bed   depth   (see  Table   18.2).     Increasing   this   grate  speed  in   an attempt to maintain a constant undergrate pressure, will then result in a reduction  of  the  clinker  residence  time  in  the cooler,  as  shown  in  Table 18.2, to  17.2 min.   This  example  shows  that by  pushing  the hot clinker  at a faster rate through   the cooler and  maintaining  the  same specific  air-flow rate and undergrate pressure,  the clinker-discharge  temperature will increase to  a   theoretical  317  F.   The  lesson  to  be  learned  from  these  examples  ; that whenever  a significantly  larger amount of clinker  falls into the cooler and as a consequence  the grate drive speed increases  significantly, the operator must compensate for this with a corresponding increase in the specific, air-flow  rate  (SCFM).

other  influencing  factors  that  cause  the  undergrate  pressure  to  change are  the  temperature  of  the clinker  in the cooler  and  the  amount  of the introduced into the individual undergrate compartments.  Assuming other factors  to  be  constant   a  higher   temperature  of   the  clinker  results   in higher undergrate pressure and increased air-flow rates in the undergrat, compartments  yield  higher  undergrate pressures.

Clinker residence time in the cooler can be determined on a theoretical basis when certain factors are known.  For  the  purpose  of  illustration, three different cooler operating conditions will now be considered to determine the effects of changes of these critical variables.

Residence  Time  at  Cosntant  Output.    Under  these  circumstances,  the bed depth is directly proportional  to the grate speed. Knowing the area of the cooler, the bed depth under normal  operating conditions  for a given kiln output rate, and the density of the clinker, the residence time can then be calculated by Eq. (18·1).

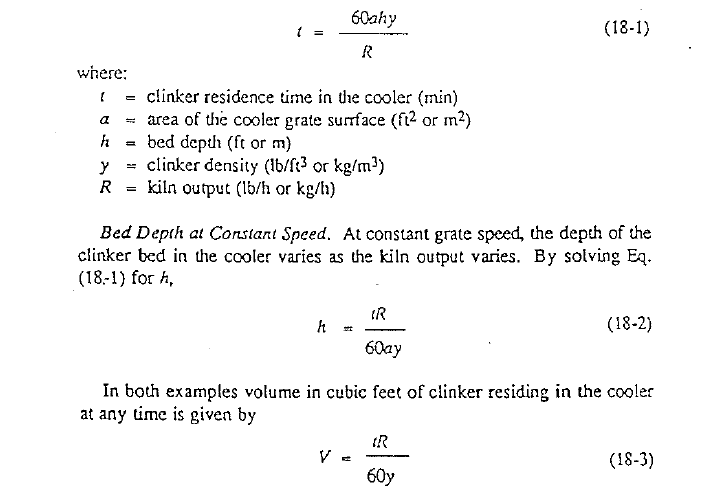
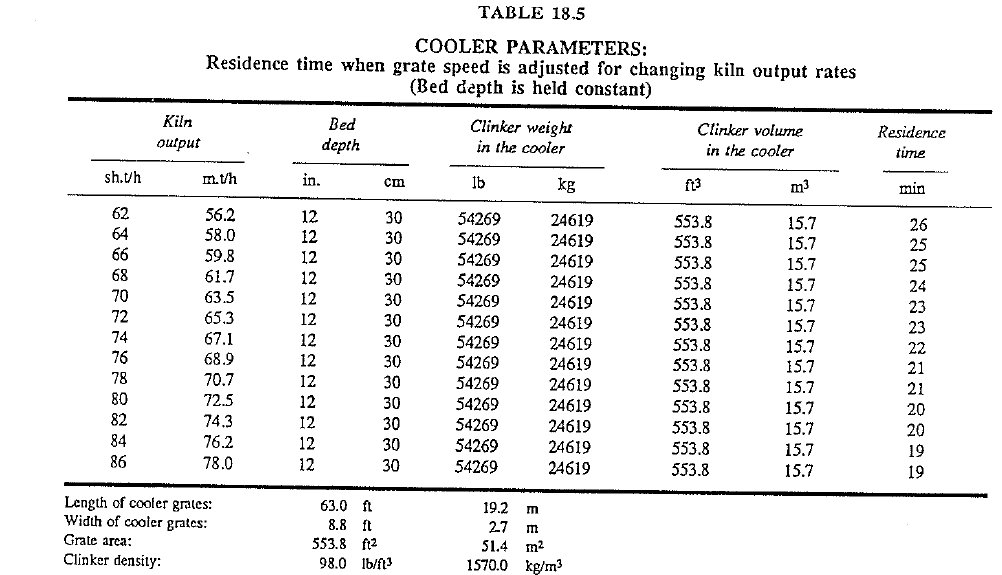
Residence Time ac Conscant Bed Depth. As mentioned previously, it is not desirable to maintain a constant undergrate pressure under all kiln out· put rates. This, of course, requires a constant bed depth, which means that the clinker residence time must be changed as the kiln output changes. Eq. (18·1) is used

An operator should have no trouble in finding solutions for  tl1e  par­ ticular cooler he is operating, as the constants in the preceding equations can be substituted so that they apply to his cooler  and kiln  operation,  and can be developed  into tables  similar to Tables  18.3, 18.4, 18.5, and  18.6.

the reader’s attention  is especially directed to Table !8.5 which clearly shows the extremes that residence time can span at kiln output rates not uncommonly obtained during upset operating  conditions.  It  also  substantiates the statement that hot clinker-discharge temperatures can become a reality  if insufficient  time is allowed to cool the clinker properly.

It should now be clear that proper cooling is not obtained by  operating with a constant undergrate pressure (bed depth) at all times,  as  it  is definitely wrong to assume that undergrate pressure is a function of bed depth only. If this were so, cooler components would not burn up at  such an alarming rate as now encountered on many rotary kilns.

PARTICLE SIZE OF CLINKER

A critical factor in undcrgrate pressure rcacticns is the average particle size of the clinker in the cooler.  The reader may be familiar with the Blaine cement surface-testing procedure in which measurement is made of the  time  required  to  pass   a  specified  amount  of  air  through  a  standard sample of cement.   It takes longer for the air to pass  through  a fine sample than a coarSe one because the finer material imposes greater resistance against  the  air.   Exactly  the same action  takes  place  when  the  clinker in the  cooler  gets finer  because  of  some  upset  kiln  operating  condition.    A finer clinker bed imposes more resistance against  air flow, undergrate pres· sure increases, and the  fan has to use more force to push  tl1e  air through this kind of a bed than through  a normal  one.  This  however  is not  the only problem Individual clinker particles are lighter when the particle size distribution Such particles can easily be lifted into the air stream above the cooler grates because of their lighter weight.

From this it becomes clear that there are two changes in the cooler, one allowing the  other.  first  the  undergrate  pressure  increases  when  the clinker gets finer because the smaller particles impede air flow through the bed. Then, when the air flow rate is increased to restore the normal no\v of air through the bed, the clinker bed can become fluidized.  As soon as this  takes place,  resistance of  the bed  to the now  of air decreases and the undergrate pressure will suddenly drop. A fluidized clinker bed is a highly undesirable and dangerous condition because a bed in such a state usually does not move along properly in the cooler. On a horizontal grate cooler the clinker bed when fluidized tends to remain stationary with more and more clinker building up on it. Then when sufficient weight has been acquired by the bed and it starts to move again, there is so much clinker present in the cooler that it cannot be properly cooled, and it could again choke off air flow through the bed, starting the cycle over again.

It is a fundamental  rule of  traveling  grate cooler operation  never  to permit raw feed or extremely fine clinker to enter the cooler. The most modem traveling grate cooler in use today is not designed to handle such fine materials and can become overheated and damaged if called upon to do so. The best procedure in such an instance is to lower the kiln speed in order that the load in the cooler is lessened, thus giving enough time for the fine clinker .to cool properly.

Another question which has to be addressed is: “How does the clinker­ discharge temperature react when the kiln output remains the same but the clinker bed depth in the cooler is increased?” Such an increase in bed depth is usually the result of:

1. deliberate decrease in grate speed due to the selection of a higher undergrate-pressure   setpoint  or
2. a design change  where  the effective grate  area of the cooler  is reduced.

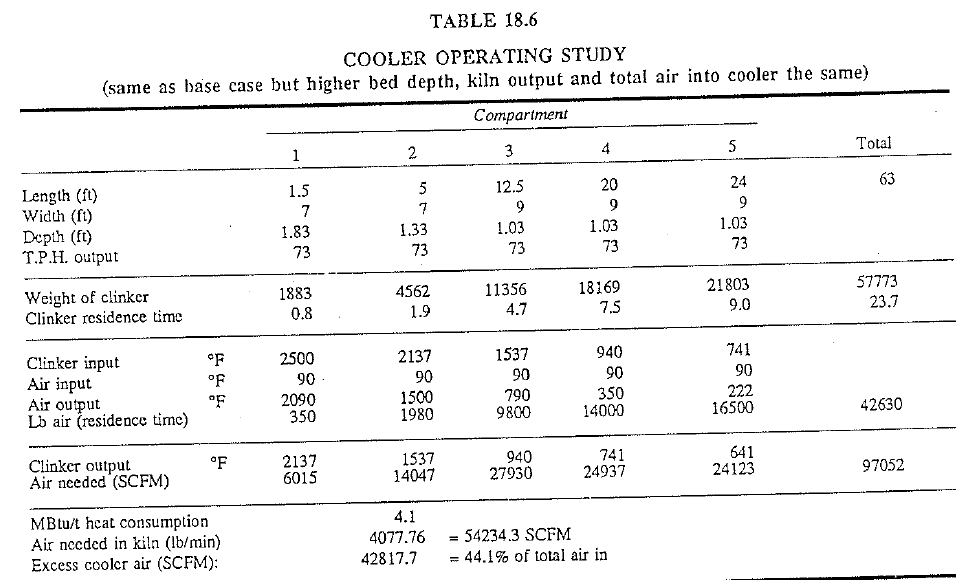
Naturally, this increase in bed depth (undergrate pressure) immediately im­ poses a higher restriction on the cooler air fans and demands sufficient fan capacity to overcome this added restriction. Consider the base case (Table 18.1). Here, 42,630 lb of air are given to the cooler in 20.9 min to cool 50,893 lb of clinker. Assume that this cooler would be operated with a higher bed depth but with the same kiln output and 42,630 lb air input. This operating condition is shown in  Table 18.6 and indicates that the clinker residence time has increased from 20.9 to 23.7 min. due to the increase in residence time, the 42,630 lb of air now represents a specific air­ flow rate of only 97,052 SCFM. The most  important  aspect  of  this change is that the resultant increase of the clinker-discharge temperature from 224 F to 641 F is completely unacceptable and dangerous. Here too, the solution is to increase the specific air-flow rate (SCFM) to compensate for the increase in bed depth.

OPERATION  OF COOLER  FANS

Now consider control of the rate of air flow in the cooler. The prime requirement in cooler control is to make sure that the air flow through the clinker bed is never restricted completely, because such a restriction leads directly to insufficient cooling of the clinker and possible damage to the cooler components. It has been pointed out that different clinker beds exert different resistances against the cooler fans.· To be able to understand this important fact more clearly, the operator must have some knowledge of how a fan works under actual operating conditions.

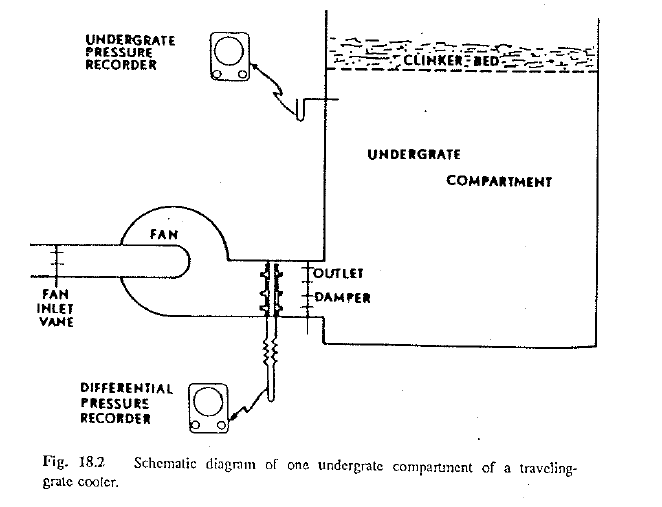
Fig. 18.2 is a simplified illustration of an undergrate compartment with its corresponding air fan. Air-volume control for cooler fans is most com­monly carried out by means of either fan outlet dampers, fan inlet vanes, or sometimes both. The fan speed is constant for any one cooler fan so it is necessary for the operator to change the position of the damper or vane to reduce or increase the volume of air moved by the fan assuming no change in static pressure on the fan.

Fan manufacturers usually supply performance curves of individual fans for the customer. Fig. 18.3 is such a curve showing the volume of air plotted against horsepower and static pressure. In order that this discussion does not become too technical and enter fields over which an operator has no control, the horsepower curve will be disregarded and attention directed to the static pressure curve.

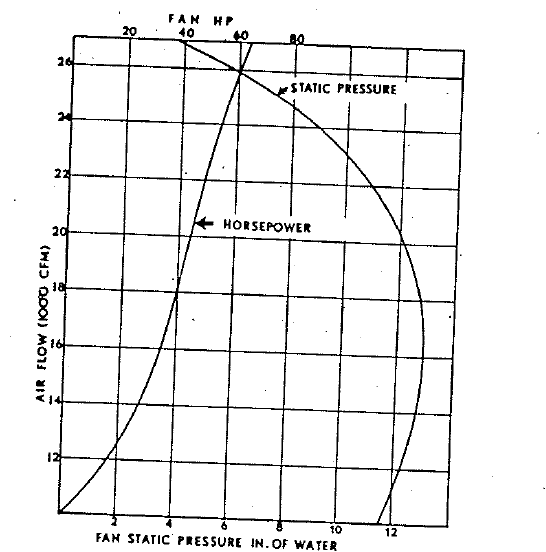
Fan static pressure is the total pressure developed by the fan, less the velocity pressure in the fan discharge duct For all practical purposes, fan static pressure in a cooler installation is equal to the undergrate pressure, within close tolerances.  Air flow is a function of static pressure and power applied to the fan. To make this discussion more meaningful, substitute undergrate pressures for equal units of fan static pressures, and restrict the range of pressures to values as they are encountered normally on rotary. kiln coolers, thus obtaining new fan performance curves as shown  in Fig. 18.4.  The curve on   the  right represents  the same curve as  shown  in Fig. 18.3, that is, the dampers of the fan are wide open, and the air flow is the maximum obtainable for this particular fan. This curve shows clearly how the air volume decreases as the undergrate pressure increases. For example, when the undergrate pressure is 9 in. of water with the damper wide open, 1e volume of air moved by the fan is 24,000 CFM: If the undergrate pressure increases, perhaps as a result of increased kiln output, the volume of air progressively decreases until at 12 in. undergrate pressure the volume is only 20,000 CFM. With the fan dampers wide open, an increase in undergrate pressure causes less air to pass through the clinker bed, creating the dangerous possibility of an overheated cooler and at the same time insufficient cooling of the clinker.

During normal and stable operating conditions, tl1e fan dampers  or vanes are never fully open. In Fig. 18.4 additional curves have  been plotted showing inlet vane openings of 90, 80, 70, and 60%. Usually, a cooler fan is operated at approximately 60% opening, thus giving the operator the necessary freedom to increase air flow if a kiln upset should make it necessary. For example, assume that the cooler is operating in a stable fashion with adequate cooling of the clinker at an undergrate pressure of 7 in., fan inlet vane at 60% opening, and air volume of 21,000 CFM. For some reason, t e undergrate pressure increases to 11 in. In order to obtain the same volume of air through the clinker bed,. the fan inlet vane opening must be increased from 60% to 90%. If the  vane  were  not opened, the air volume would drop to 19,000 CFM.

These examples of fan performance  under actual operating conditions point out two significant facts: First, as with any butterfly-type valve or damper, maximum flow is reached when the damper is about 88% open. Any enlargement in the opening thereafter gives only a very small increase in air flow. Second, air-volume output is directly related to undergrate pres­sure. Therefore, the fan must have sufficient capacity to provide the necessary amount of air at the maximum anticipated  undergrate pressure.

A word of caution must be introduced here. Each fan installation will have its own characteristics. The fan curves discussed in this chapter apply to one certain installation and can be considered as typical, to show the reader the relationships between pressure, output, and power requirements. Reactions and capacities of any individual-installation must be computed for that particular combination of equipment. For this reason, it is advis­able for an operator to examine the curves of the particular cooler fans that are under his control, and become familiar with their characteristics and capacities, so he will know the operating limits for undergrate pressure. Whenever the undergrate pressure exceeds these limits, the operator will know then that less efficient cooling is taking place and that an immediate change in kiln speed must be made to avoid damage to the cooler.

CLINKER AND AIR DISTRIBUTION THE COOLER

Finally, it is necessary to consider distribution of clinker and air in the cooler. For proper cooling of the clinker it is essential that the clinker is evenly spread over the width of the cooler so that the bed offers uniform resistance to the passage of air throughout its width. When the clinker passes to one side of the cooler, leaving a thinner bed on the opposite side, the air. will naturally seek a passage through the bed where it offers the least resistance. Consequently, the air passes through the bed where it is least needed and little air passes where it is needed most. Formation of stalagmites (commonly referred to as “snowmen” or “candles”) at the cool­er inlet is the prime cause of this condition. Various devices are ·used to combat stalagmite formations. Some coolers  have  water cooled  steel jackets, or water cooled clinker spreaders, and others have a special row of quench grates with their own air supply, to spread the clinker rapidly over the width of thee cooler at the inlet.

For proper distribution of air in the cooler no air should  freely pass from one undergrate compartment to another through large leaks or other openings. If this is allowed  to take place, the air introduced, for example into the first compartment, could pass over into the second compartment when the clinker bed is thicker at the cooler inlet.

Proper clinker distribution  is an acute problem  in tl1e upper region of many grate coolers.  This problem can usually be overcome by:

1. narrowing tl1e  cooler  width  by  means  of  installing  refractory ledges and or “dead” grates along the cooler walls and
2. concurrently increasing  the  static-pressure  capability  of  the  air fans in these narrowed

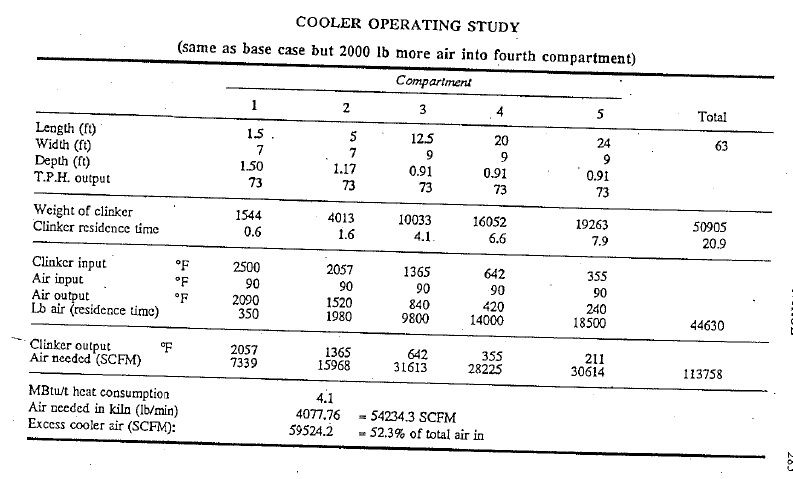
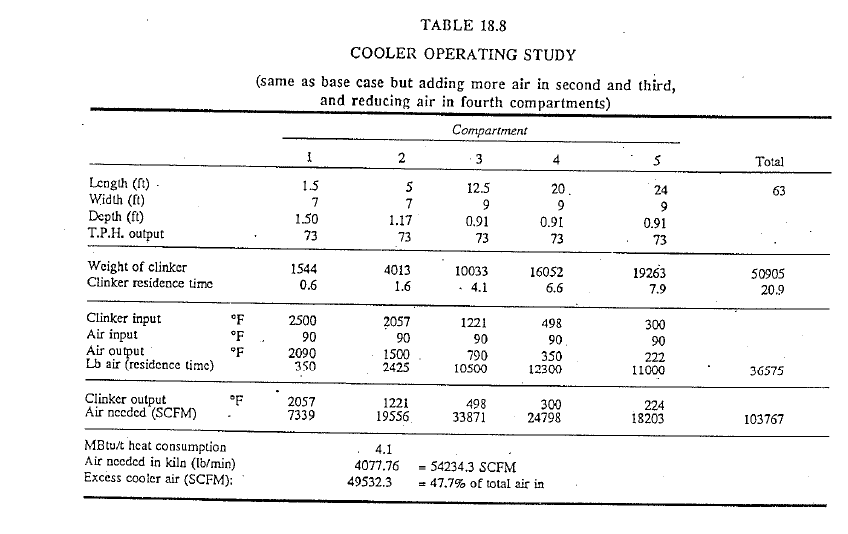
In Chapter 12 it was brieOy mentioned how important.it is to apply the maximum air in these compartments where it can do tl1e most thermal. work.  This means, tl1e majority of the cooling work should be accom­ plished in the first and second compartments because it is here that the most efficient heat transfer will take place due to the large temperature difference between the air and the clinker.  Once again, the base case in Table 18.1 is considered and it is assumed that 2000 lb more air is added into the fourth cooler compartment, i.e.; at the .place in the cooler where heat transfer is relatively ineflicient.   Table 18.7 shows that this 4.7% increase in total air input results in a clinker-discharge-temperature drop of only  13 F (from 224 F to 211 F).  It is assumed that all compartment air flows are readjusted to take advantage of the aforementioned thermo­ dynamic principle. In Table 18.8 air has been added to the second and third compartments while it has been reduced to the fourth and fifth compart­ments.   The end result  is that the total air input into the cooler has been  reduced by 15% but the clinker-discharge temperature remains the same at 224 F. Once again, it is important for an operator to remember  this principle of cooler-air application as this will lead to efficient cooler operation and possibly to less failures and damages to cooler components.

Undergrate pressures are usually set so that the highest pressure is found in the first compartment and the lowest in the last compartment. Clinker temperatures above the grates, as pointed out earlier, influence the under­ grate pressure. This step-by-step lowering of the undergrate pressures from one compartment to another is a natural result of the succeeding lower temperaures of the clinker as it travels down the cooler.

SECONDARY AIR-TEMPERATURE CONTROL

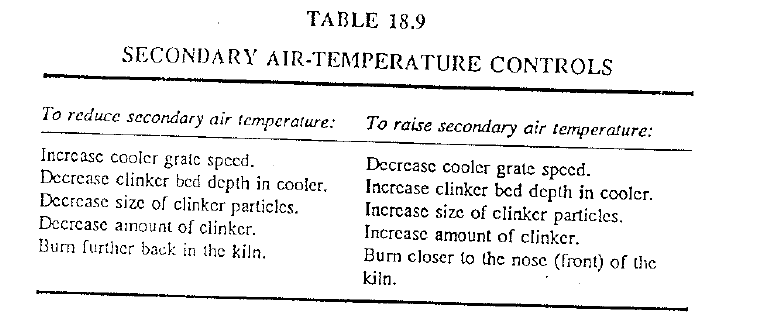
Secondary air temperature has a direct influence on the geometry of the flame and the point of ignition of the fuel, consequently irregular secondary air temperatures can cause irregular flame characteristics which in turn can cause a shifting of the burning zone. Stable kiln conditions are practically impossible as long as the secondary air temperature is not held constant, within a tolerance of± 100 F (40 C). The following discussion applies to controls to be exercised when the kiln is operating at normal speed and is producing well-burned clinker.

The kiln should be operated with the secondary air temperature as high as possible because the maximum amount of heat is then recovered from the clinker, thus improving fuel efficiency, as less fuel is required to raise the temperature of the air entering the kiln. This condition maximizes kiln capacity. A second advantage of high secondary air temperature is the favorable influence on the objective of burning the clinker close to the nose (front) of the kiln. However, there are practical limits for secondary air temperatures. A temperature that is too high can result in overheating in the kiln nose and the burner hood as well as in the cooler. It is advisable to operate the kiln with the secondary air temperature slightly below the maximum allowable in order to protect the bed grates and cooler refractory from damage.

The two factors having the greatest influence on secondary air temperature are speed of the bed grates in relation to the volume and temperature of air introduced into the cooler to cool the clinker, and temperature and size  of clinker discharging from the kiln into the cooler. Under stable kiln con­ditions, the secondary air temperature is controlled by the speed of the cooler grates which merely means that the depth of the clinker bed in the cooler is the controling factor. Thus, an increase in grate speed (lessened bed depth), other conditions remaining unchanged, results in a lower secon­dary air temperature, and a slower grate speed (thicker bed depth) causes an increase in temperature. It is important to remember, however, that secon­dary air temperature control is not merely  a matter of speeding up or slow­ing down movement of the bed grate. As  mentioned  earlier,  there  are several factors to be considered before deciding which adjustment will give the desired results.

Because the secondary air temperature is controlled mainly by the  bed­ grate speed and the volume and temperature of the air in the cooler, as well as the temperature and size of the clinker,  this  control  goes hand  in  hand with undergrate-pressure control, as any change in these two variables will also change the undergrate pressure. This then will considerably limit the extent to which  secondary  air  temperature  can  be controlled.

Now consider for example, an upset kiln condition in which the greatest part of the material in the cooler  is  in  the  form  of  very  small-sized  nod­ules, or even worse, in the form of dust. In a situation like this the operator will first reduce  the kiln speed, which  reduces the amount of clinker and consequently lowers the secondary air temperature (see Table 18.9). If an attempt is made to hold the secondary air temperature within a 100-degree tolerance, it would  be necessary  to slow down  the bed-grate  speed to such an extent that it would choke  off  the  free passage  of  air through  the  bed. The clinker would thus not  be properly  cooled  and would  probably be red hot on leaving the cooler, causing considerable damage to the clinker trans­port equipment. The proper  adjustment  in  this  case  would  be  to  slow down the bed grates  only  to such  an extent  that the normal  bed  depth can be maintained. It is also extremely important  that  the  air  flow  into  the cooler be increased in order that the clinker can be properly cooled. The operator should never attempt to hold the secondary air temperature  at  its normal level when  the kiln  has been slowed down because of an upset.

The other factor to be considered in secondary air-temperature control is the temperature of the clinker as it discharges from the kiln. By changing the character of the name, the burning zone can be shifted closer to the kiln front thus raising the clinker-discharge temperature and consequently the secondary air temperature, or the burning zone can be shifted further back in the kiln, reducing the secondary air temperature. These actions are summarized in Table 18.9.

Earlier in this chapter  it was pointed out that more air must always be given to tile cooler than  what is needed for combustion  in the kiln.  Hence, a certain amount  of  excess  air has  to  be  vented  to  the  atmosphere  or  used for the drying and grinding of raw materials and/or coal.  On precalciner kilns, this excess air is diveided to the flash calciner by means of the ter­tiary air duct. By inference there must therefore be an imaginary dividing line in the cooler wherein the air in the hotter region goes to the kiln  and the one in the colder part moves toward the cooler stack. Simple calcu­lations on  the operating conditions explained in  Tables  18.1  and  18.8 would show that more efficient application of the air in the first two compartments would result in a shift of this  imaginary  line  toward  the upper end of the  cooler, higher secondary air, and lower cooler-stack air temperatures.

Design limitations, either in cooler size or fan capacities, often lead to excessively high clinker-discharge temperatures regardless of how well the air distribution is controlled or the cooler mechanically maintained. Such kilns are usually capable of producing more clinker but the cooler acts as the bottleneck toward these higher output rates. Short of installing an after-cooler (such as the well-known G-cooler) or major modifications in cooler design, there is not much one can do to overcome these limitations. Some plants use water-spray cooling of the clinker within the last cooler compartment (usually leading to operating problems with the dust collec­tor) or make use of reciprocating clinker “skips” combined with water sprays after the clinker discharges from the cooler. These solutions must be viewed as only temporary as most of these create additional operating problems.

HOOD-DRAFT  (PRESSURE) CONTROL

A defmitive volume of air is drawn into the kiln by the !.D. fan and a given amount of air is forced into the cooler by the cooler fans. Years ago, on wet-process kilns with their high specific-heat consumption and relatively low kiln output, these two flows were nearly equal. Hence, only small amounts of excess air had to be vented to the atmosphere by means of the cooler stack. Some of these kilns were  equipped with so-called closed-circuit cooler-air systems (recycling excess air to the upper cooler compartment fans) and successfully eliminated all excess air.  However, as kilns became more efficient in heat consumption and clinker output rates, excess cooler-air volumes started to increase and in many cases this led to difficulties in hood-draft control. All too often the damper in the cooler stack which regulates the hood draft is found to be in the fully  open position thus eliminating any effective control of the hood draft.

Hood-draft  control is simply a regulation  of the amount of excess air which escapes through the cooler chimney, so that when the hood pressure is too high, the damper must be opened, and when the hood pressure is too low, the damper must be closed. This does not mean that the damper has to be fully closed or fully opened, but instead small  adjustments in the damper position can be made to give the desired results.

As mentioned, hood draft is governed mainly by:

1. the D. fan speed and
2. the volume of air given to the cooler

Assuming that other factors remain constant, then an increase in J.D. fan speed results in a lower hood pressure, and a reduction in I.D. fan speed results in a higher hood pressure. Similarly, increasing the amount of ak forced into the cooler results in higher hood pressure.

The hood pressure can be either negative or positive.  A negative pres­sure indicates that the hood is under a vacuum,  and a positive hood pres­sure means that the hood is pressurized.   The basic rule in hood-pressure control is never to operate a kiln with positive hood pressure because this results in troublesome kiln operating conditions.  Fine clinker particles are blown  through  the nose-ring  seal thus causing  the seal to wear out pre­maturely,  and dust emission  in  the  hood  area can  make  viewing  of the burning  zone  by  the  operator  unpleasant  and  unsafe.  On rotary kilns equipped with optical pyrometers and television cameras for burning-zone control, positive hood pressures could lead to damage to this  equipment from the flying hot particles. Formation of rings and of stalagmites (“snowmen”) in the”cooler inlet can be attributed to positive hood pres­sures on some kilns. The importance of  operating  a kiln  with  negative hood pressure is obvious.

There is one situation in which the operator has to take exception to the above  rule:  Whenever  prevailing  high  cooler  temperatures  could  cause damage to the cooler components, it is necessary to introduce a sufficient amount of air into the cooler to lower the temperature and overcome the dangerous situation. The first corrective action in such an instance would be to slow down the kiln speed to lessen the load in the cooler. Then if dangerous overheated conditions still prevail and the damper at the chimney is already wide open, one has no other choice but to introduce an added vol­ume of air into the cooler. However, the operator should return the !load pressure to negative again at the earliest possible time as soon as the situation has been brought under control.

The hood pressure is usually automatically controlled. The controller receives an input signal from the hood-pressure measuring instrument and sends an output signal to the damper at the cooler chimney. The operator adjusts the setpoint on the controller to the desired hood pressure (generally between –D.07 and –D.03 in. of water). Any time the hood pressure deviates from this setpoint, the chimney damper will then be automatically adjusted by the controller.

click here to Download the Most Important 13 Books in Cement Industry 

click here to Download the Most Important 13 Books in Cement Industry